Block Diffusion

BD3-LMs: Block Discrete Denoising Diffusion Language Models

We combine modeling paradigms to enjoy better likelihoods & flexible-length generation from autoregressive models, as well as fast & parallel generation from diffusion models.

Block Diffusion Likelihood

We propose a modeling framework that autoregressively models blocks of tokens and performs diffusion within each block. Our likelihood factorizes over \( B \) blocks of length \( L’ \) as

\[ \log p_\theta (\mathbf{x}) = \sum_{b=1}^B \log p_\theta (\mathbf{x}^b | \mathbf{x}^{\lt b}) \]

Each \( p_\theta (\mathbf{x}^b | \mathbf{x}^{\lt b}) \) is modeled using discrete diffusion ELBO over a block of \( L’ \) tokens. We obtain a principled learning objective \( \mathcal{L}_\text{BD}(\mathbf{x}, \theta) \) by optimizing the following likelihood bound:

\[ \log p_\theta(\mathbf{x}) \geq \mathcal{L}_\text{BD}(\mathbf{x}, \theta) := \sum_{b=1}^{B} \mathcal{L}_{\text{diffusion}}(\mathbf{x}^b, \mathbf{x}^{\lt b}, \theta), \]

We model the per-block likelihood under a simple discrete diffusion parameterization (Sahoo et. al, Shi et. al, Ou et. al). Our final objective becomes a sum of weighted cross-entropy terms:

\[ \mathcal{L}_\text{BD}(\mathbf{x}, \theta) := – \sum_{b=1}^{B} \mathbb{E}_{t \sim [0, 1]} \mathbb{E}_{q} \frac{1}{t} \log p_\theta(\mathbf{x}^b | \mathbf{x}_{t}^b, \mathbf{x}^{\lt b}) \]

Efficient Training & Sampling Algorithms

Naively, we would compute the logits by applying \( \mathbf{x}_\theta^b( \mathbf{x}_t^b, \mathbf{K}^{1:b\text{-}1}, \mathbf{V}^{1:b\text{-}1}) \) in a loop \( B\) times. Instead, we only require two forward passes. The first pass precomputes keys and values \( \mathbf{K}^{1:B}, \mathbf{V}^{1:B} \) for the full sequence \( \mathbf{x}\). In the second forward pass, we compute denoised predictions for all blocks simulatenously using \( \mathbf{x}_\theta^b( \mathbf{x}_t^b, \mathbf{K}^{1:b\text{-}1}, \mathbf{V}^{1:b\text{-}1}) \).

To sample from BD3-LMs, we generate one block at a time, conditioned on previously sampled blocks. After generating a block, we cache its keys and values, similar to AR. We may use any diffusion sampling procedure \( \text{SAMPLE} ( \mathbf{x}_\theta^b, \mathbf{K}^{1:b\text{-}1}, \mathbf{V}^{1:b\text{-}1}) \) to sample from the conditional distribution \( p_\theta (\mathbf{x}_s^b | \mathbf{x}_t^b, \mathbf{x}^{

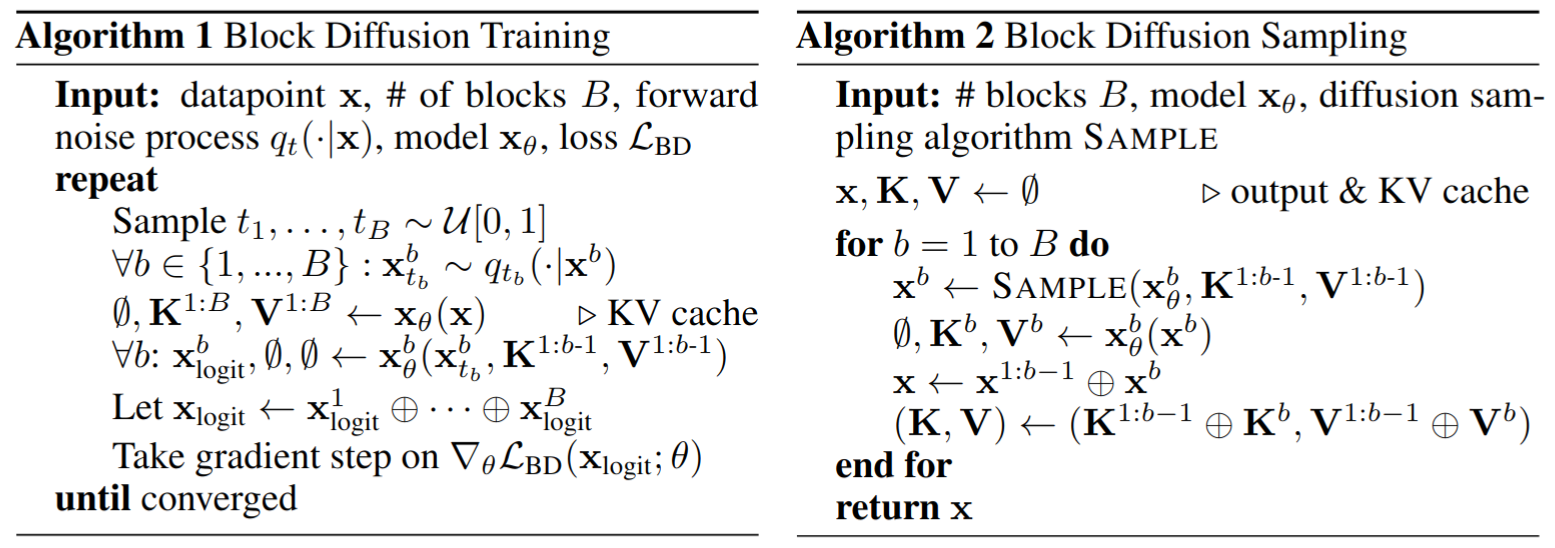
BD3-LM training and sampling algorithms.

Keep your files stored safely and securely with the SanDisk 2TB Extreme Portable SSD. With over 69,505 ratings and an impressive 4.6 out of 5 stars, this product has been purchased over 8K+ times in the past month. At only $129.99, this Amazon’s Choice product is a must-have for secure file storage.

Help keep private content private with the included password protection featuring 256-bit AES hardware encryption. Order now for just $129.99 on Amazon!

Help Power Techcratic’s Future – Scan To Support

If Techcratic’s content and insights have helped you, consider giving back by supporting the platform with crypto. Every contribution makes a difference, whether it’s for high-quality content, server maintenance, or future updates. Techcratic is constantly evolving, and your support helps drive that progress.

As a solo operator who wears all the hats, creating content, managing the tech, and running the site, your support allows me to stay focused on delivering valuable resources. Your support keeps everything running smoothly and enables me to continue creating the content you love. I’m deeply grateful for your support, it truly means the world to me! Thank you!